Understanding precision, accuracy, and tolerance of geospatial datasets: an example based on the usage of GNSS receivers

How GNSS work

This text is mainly extraxted from An Introduction to GNSS by Hexagon | NovAtel

A bit of history

Global Navigation Satellite Systems (GNSS) started with the launch of the U.S. Department of Defense Global Positioning System (GPS) in the late 1970s. Early applications of GNSS were developed for the military and soon expanded to the survey and mapping industries – driven largely by the tremendous advances in accuracy, efficiency as well as cost reductions. Now, vehicles, whether on land, in the air or at sea, routinely rely on the precise positioning information provided by GNSS technology.

The basic concept of GNSS positioning

A simple demonstration, conceived for elementary school childrens, can be used to understand how GNSS positioning works. You tack cardboard figures of three satellites to the walls and ceiling of a classroom: each “satellite” has a length of string stapled to it. You mark a location on the floor with a movable dot, then drew the strings down and marked where they all reached the dot. The strings now represented the distances from the dot to the individual satellites (Figure 2). You record the location of the dot and removed it from the floor.

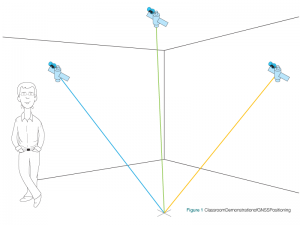
Then, the students are asked to use the strings to determine the location. To do this, the students drew the strings down until the ends of the strings came together at one point on the floor. They mark this point with a movable dot and compare it with the previously marked position. The two points will be close to each other. This simple demonstration showed that, if you know the location of three satellites and your distance from them, you can determine your position.

To sum up, GNSS positioning is based on a process called trilateration. Simply put, if you don’t know your position, but do know your distance from three known points, you can trilaterate your location. A receiving antenna measures the distance to the satellites above its horizonKnowing the distance to at least 3 satellites and applying the trilateration method, it is possible to derive the geographical position of a point (Figure 3).

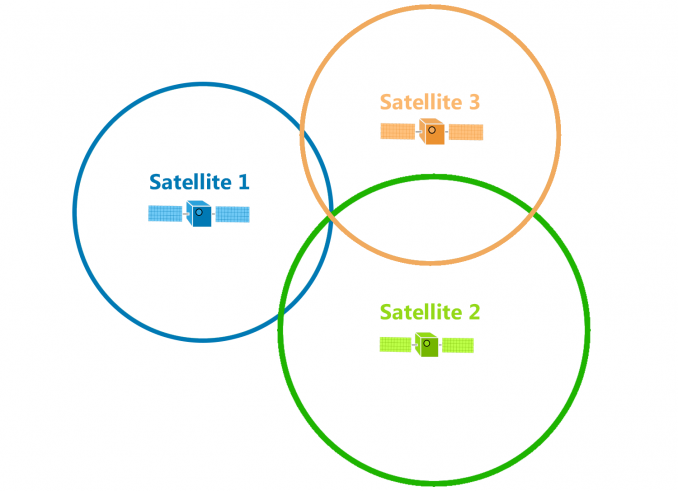
But the reality is in our three-dimensional world that GNSS satellites broadcast signals as a sphere. Each satellite is at the center of a sphere. Where all spheres intersect determines the position of the GPS receiver.

The determination of position is made quite a bit more complicated by several factors: the satellites are moving, the signals from the satellites are very weak by the time they reach the earth, the atmosphere interferes with the transmission of radio signals and, for cost reasons, the user equipment is not as sophisticated as the equipment in the satellites. 

For a more in depth description of GNSS positioning, please refer to An intuitive approach to the GNSS positioning ESA article.